The Reynolds number (Re) is a dimensionless quantity used in fluid mechanics to characterize the flow regime of a fluid. It is named after Osborne Reynolds, a pioneering scientist in fluid dynamics. The Reynolds number is defined as the ratio of inertial forces to viscous forces within a fluid flow system and is expressed as:
Re=ρ⋅V⋅L/μ
Where:
- ρ is the density of the fluid,
- V is the characteristic velocity of the flow (such as the velocity of the fluid relative to an object),
- L is a characteristic length (such as the diameter of a pipe or the chord length of an airfoil), and
- μ is the dynamic viscosity of the fluid.
The Reynolds number provides valuable information about the flow behavior:
- For low Reynolds numbers (typically less than 2000), the flow is laminar, characterized by smooth, ordered flow with little mixing.
- For moderate Reynolds numbers (between 2000 and 4000), the flow is transitional, meaning it exhibits characteristics of both laminar and turbulent flow.
- For high Reynolds numbers (greater than 4000), the flow is turbulent, characterized by chaotic, highly mixed flow patterns.
The Reynolds number is widely used in engineering and fluid dynamics to predict flow phenomena, determine flow regimes, and scale model experiments. It helps engineers design and analyze systems such as pipes, pumps, aircraft, and ships by understanding the dominant forces affecting fluid flow.
Understanding the Reynolds Number
Title: Understanding the Reynolds Number: Bridging Fluid Dynamics and Practical Applications
The Reynolds number, a dimensionless parameter introduced by Professor Osborne Reynolds in his seminal studies on fluid flow dynamics, revolutionized our understanding of how fluids behave in various conditions. Born out of experiments with a mixture of water and glycerin, Reynolds observed the transition of fluid flow from laminar to turbulent, a distinction that paved the way for numerous applications across industries.
At its core, the Reynolds number (Re) serves as a metric to quantify the balance between inertial and viscous forces within a flowing fluid. It is defined as the ratio of inertial forces to viscous forces, given by the formula:
Re=ρVD / μ
Here, ρ represents the density of the fluid, ( V ) denotes the mean velocity, ( D ) stands for the characteristic dimension (typically the diameter of the pipe), and ( \mu ) symbolizes the dynamic viscosity of the fluid.
Laminar flow, characterized by smooth and ordered fluid motion, prevails at low Reynolds numbers where viscous forces dominate. In contrast, turbulent flow emerges at high Reynolds numbers, where inertial forces dominate, leading to chaotic eddies, vortices, and flow instabilities.
In practical applications such as aerodynamics, the Reynolds number plays a pivotal role, especially in understanding airflow around objects like wings. For instance, in aerodynamic analyses, the chord of the airfoil (( c )) is often used instead of the pipe diameter (( D )), reflecting the appropriate characteristic length.
In the world of aviation, where Reynolds numbers can soar into the millions, low viscosity effects and thin boundary layers are desirable. However, as flow progresses along the body, boundary layers may thicken and eventually transition to turbulent, increasing drag.
To mitigate this, techniques like boundary layer suction have been proposed, albeit often impractical due to mechanical complexity and power requirements. Alternatively, the concept of natural laminar flow involves shaping airfoils or fuselages to delay boundary layer transition, effectively reducing drag and enhancing aerodynamic efficiency.
In essence, the Reynolds number serves as a fundamental tool in understanding fluid dynamics, guiding engineering decisions, and optimizing designs across various disciplines, from aerospace to plumbing, and beyond. Its legacy continues to shape modern advancements, driving innovation and efficiency in fluid-related endeavors.
How to Calculate Reynolds Number?
Let’s consider a scenario where we want to calculate the Reynolds number for water flowing through a pipe with a diameter of 0.1 meters (100 millimeters). The water velocity is 1 meter per second, and the dynamic viscosity of water at the given temperature is 1×10−31×10−3 Pa·s.
Given:
- Density of water (ρ): 1000 kg/m³
- Mean velocity (V): 1 m/s
- Diameter of the pipe (D): 0.1 m
- Dynamic viscosity (μ): 1×10-3 Pa·s
We can use the formula for Reynolds number:
Re=ρVD / μ
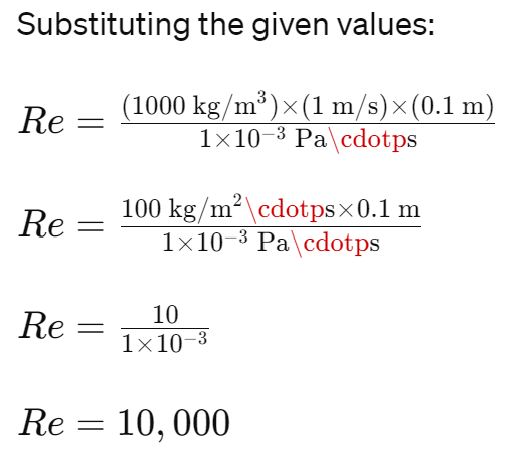
So, the Reynolds number for this scenario is 10,000. This indicates that the flow is in the turbulent regime since it exceeds the critical value for laminar-turbulent transition (typically around 2000).