What is Kirchhoff’s Circuit Law?

Kirchhoff’s Current Law (KCL).

Kirchhoff’s Current Law states that the total amount of current entering a junction (or node) in a circuit is equal to the total amount of current leaving the junction. In other words, whatever current flows into a point must flow out of that point.

Why It’s Important:

• This law helps us understand and calculate how current is distributed in a circuit.
• It is based on the principle that electric charge is conserved, meaning it doesn’t just disappear.

Mathematical Form:

I_entering​ + I_leaving​ = 0

Node: A node in an electrical circuit is a connection or junction where two or more current-carrying paths or elements such as cables and components meet.

Conservation of Charge: KCL is based on the conservation of charge principle, which means that the total charge is conserved in a node, with no loss of charge.

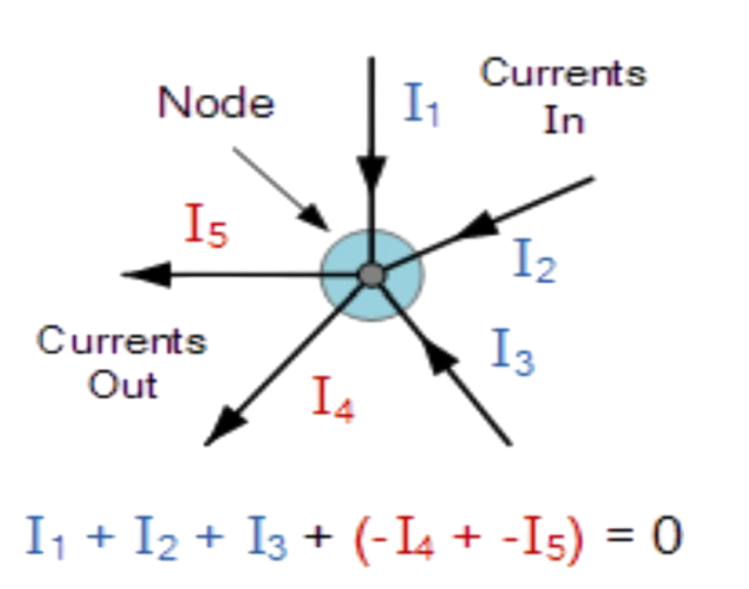
Example: Consider a node where three currents (I1​, I2​, I3​) are entering the node and two currents (I4​, I5​) are leaving the node. According to KCL, we can write the equation as:

I₁ ​+ I₂ ​+ I₃ ​−I₄ ​− I₅ ​= 0

In this equation:

Currents I₁ ​, I₂ ​and I₃​ are positive because they are entering the node. Currents I₄ ​and I₅ are negative because they are leaving the node.

Kirchhoff’s Voltage Law (KVL):

Kirchhoff’s Voltage Law states that the sum of all electrical potential differences (voltages) around any closed loop in a circuit is equal to zero. This means that the total amount of energy gained per unit charge must be equal to the total energy lost per unit charge in a closed loop.

Mathematical Form: ∑V=0.

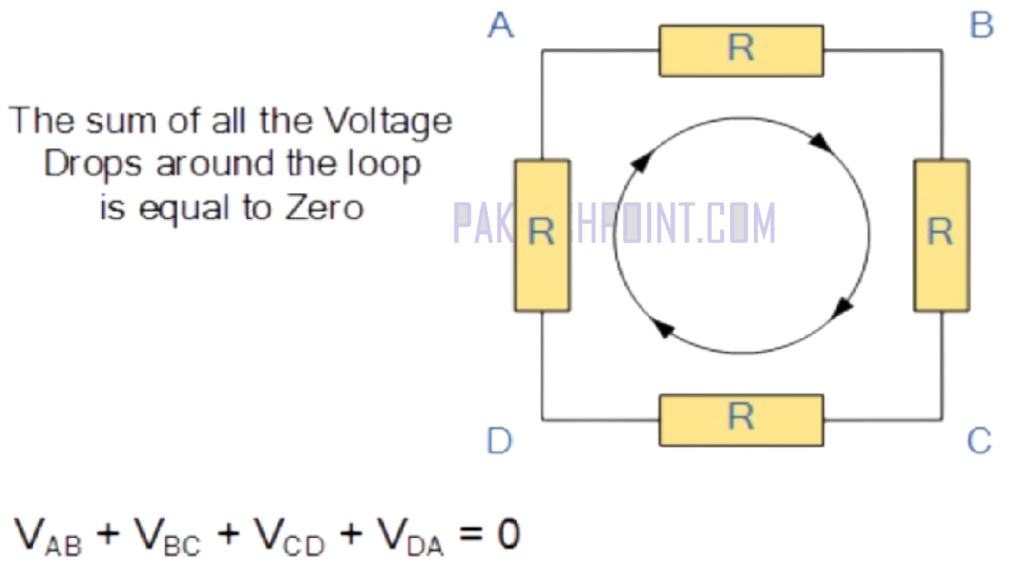
Closed Loop: A closed loop is any path in a circuit that starts and ends at the same point without crossing itself.

Conservation of Energy: KVL is based on the conservation of energy principle, ensuring that all the energy supplied to the loop is used up by the elements within the loop.

Consider a simple loop with a voltage source V and resistors R₁​ and R₂​. If the current I flows through the loop, the voltage drops across the resistors will be IR₁ ​and IR₂​. According to KVL, the sum of the voltage source and the voltage drops must be zero:

V − IR₁ ​− IR₂ ​= 0

Practical Applications

Circuit Analysis:

By applying KVL, we can determine the voltage drops across various components in a circuit. This is crucial for designing and troubleshooting electrical circuits.

Mesh Analysis:

KVL is used in mesh analysis, a method for analyzing complex circuits by creating and solving a set of equations based on KVL for different loops in the circuit.

Energy Conservation:

Ensures that the energy supplied by sources (like batteries) is fully accounted for in the circuit elements (like resistors and capacitors).

DC Circuit Terms used

Here are some fundamental terms used in DC circuit theory,

Circuit

A circuit is a closed loop conducting path in which an electrical current flows. A simple circuit with a battery and a light bulb connected by wires.

Path

A single line of connecting elements or sources.The wire connecting the positive terminal of a battery to one terminal of a light bulb.

Node

A node is a junction, connection, or terminal within a circuit where two or more circuit elements are connected or joined together, providing a connection point between two or more branches. A node is indicated by a dot.The point where three wires meet in a circuit.

Branch

A branch is a single or group of components such as resistors or a source that are connected between two nodes. A resistor connected between two nodes in a circuit.

Loop

A loop is a simple closed path in a circuit in which no circuit element or node is encountered more than once. A loop formed by a battery, a resistor, and connecting wires.

Mesh

A mesh is a single closed loop series path that does not contain any other paths. There are no loops inside a mesh. In a circuit with multiple loops, a mesh is one of the individual loops without any other loops inside it.

These terms and their definitions are basics of DC circuit theory, allowing for precise and effective communication when discussing and analyzing electrical circuits.

DC Circuit Example:

Kirchhoff’s Circuit Law Example 1.

Find the power supplied by element D and the power received by element F in the circuit, we need to follow the steps and calculations.

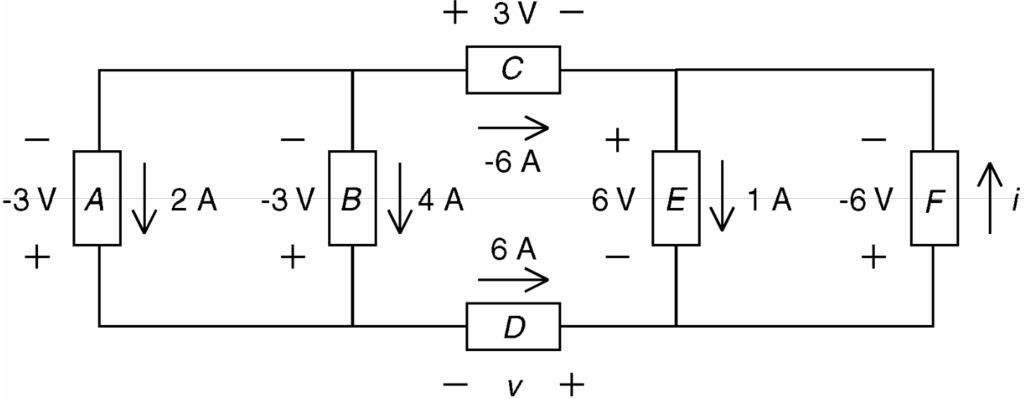
To find the power supplied by element D and the power received by element F, we need to determine the missing voltage across element D and the current through element F. We can use Kirchhoff’s laws to do this.

Element D: We have the current but not the voltage. The voltage and current do not follow the passive sign convention (to find power), so the power calculation will show the power supplied by element D.

Element F: We have the voltage but not the current. The voltage and current follow the passive sign convention (to find power), so the power calculation will show the power received by element F.

1. Use Kirchhoff’s Voltage Law (KVL):

• Apply KVL to the loop containing elements C, E, D, and B to find the voltage V across element D.
• Equation: (3 V) + (6 V) + V_D + (−3 V) = V_D = -6 V
• From Figure 1, the current through element D is 6A.

Calculate Power Supplied by Element D:

P_D = V_D x I_D =(−6 V)⋅(6 A)= −36 W

Since the power is negative, element D is supplying 36 W of power.

2. Use Kirchhoff’s Current Law (KCL):

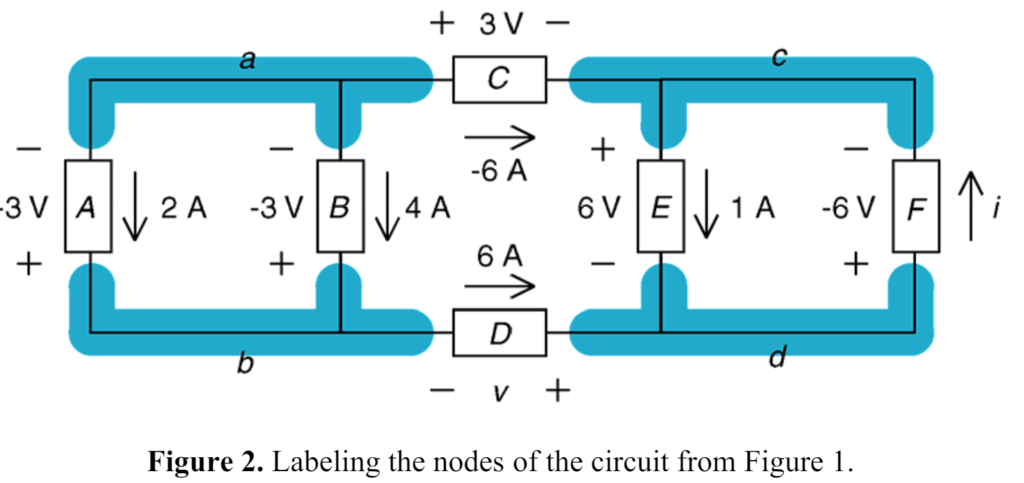
Apply KCL at node c to find the current i through element F.

-6A = 1A + I_F = I_F = -6A -1A = -7A

As we know, From Figure 1, the voltage across element F is −6 V.

Calculate Power Received by Element F:

P_F = V_F x I_F = (−6 V)⋅(- 7 A) = 42Watt

The power received by element F is 42 W.

Following document represent more examples of Kirchhoff’s Circuit Law.

KCL_KVL-Examples