What is Mesh Current Analysis?

Mesh Current Analysis, also known as Loop Analysis or Maxwell’s Circulating Currents method, is a technique used to simplify the analysis of electrical circuits, especially when dealing with complex networks.

Instead of labeling each branch with a current (Kirchhoff’s Current Law), Mesh Current Analysis labels each “closed loop” in the circuit with a circulating current. This method reduces the amount of math needed and makes solving large circuits more manageable.

Here’s a step-by-step guide to make it easier:

Mesh Current Analysis Steps

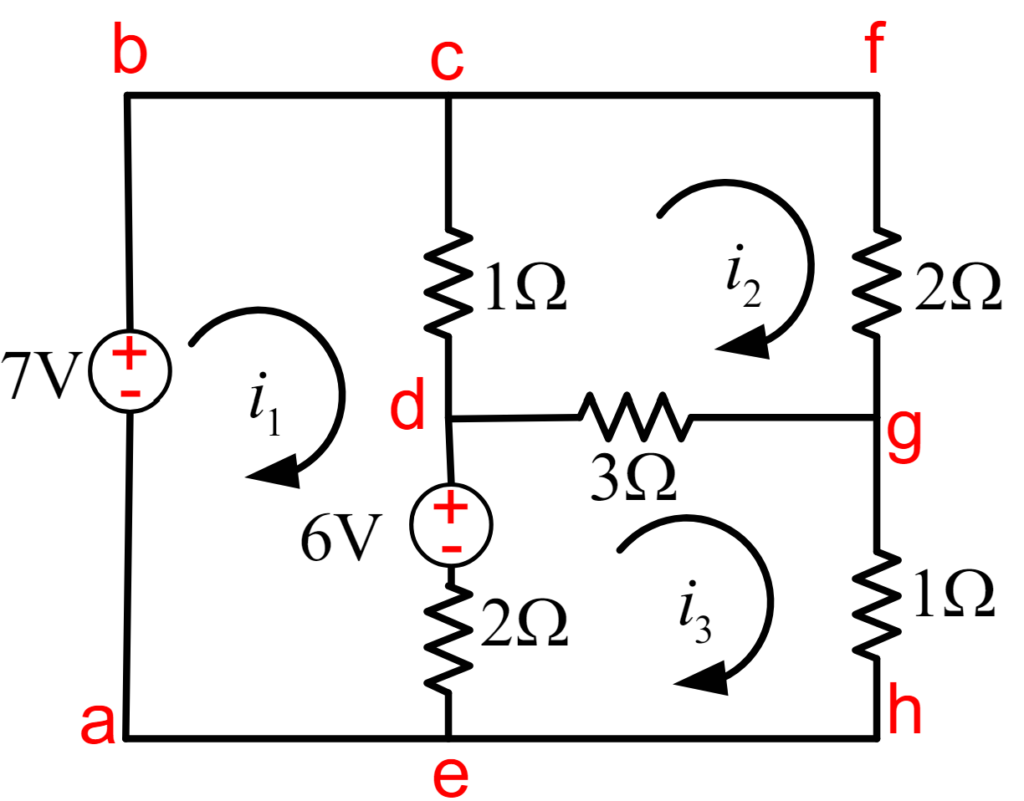
1. Identify the Meshes: A mesh is a loop that does not enclose any other loops within it. Identify all the meshes in the circuit. In above diagram abcdea is mesh.
2. Assign Mesh Currents: Assign a current variable to each mesh. The currents are usually assumed to circulate in a clockwise direction, but you can choose any consistent direction.
3. Apply Kirchhoff’s Voltage Law (KVL): Write KVL equations for each mesh. KVL states that the sum of all voltages around a closed loop is zero. Include voltage sources and the voltage drops across resistors (Ohm’s Law: V=IR) in the equations.
4. Solve the Equations: Solve the simultaneous equations by cramer’s rule or other to find the values of the mesh currents.
5. Determine the Desired Quantities: Use the mesh currents to find the voltages and currents in individual circuit elements, and calculate the power as needed.

Mesh Current Analysis Example 1

Determine the mesh currents i₁, i₂, i₃ in below circuit diagram.

Step 1: We identified all meshes of circuit as shown in above picture.

Step 2: We assigned all meshes of current which are i₁, i₂, i₃ .

Step 3: Applying Kirchhoff’s Voltage Law (KVL):

so considering the circuit loop abcdea.

1(i₁ − i₂) + 2(i₁ − i₃) + 6 − 7 = 0

3i₁ − i₂ − 2i₃ = 1 Equation-1

Now take the 2nd loop cfgdc.

2i₂ + 3(i₂ − i₃) + 1(i₂ − i₁) = 0
−i₁ + 6i₂ − 3i₃ = 0 Equation-2

Now take 3rd loop dghed.

3(i₃ − i₂) + 2(i₃ − i₁) + i₃ − 6 = 0
−2i₁ − 3i₂ + 6i₃ = 6 Equation-3

Now take a look on 3 mesh equations 1, 2 and 3.

3i₁ − i₂ − 2i₃ = 1 −i₁ + 6i₂ − 3i₃ = 0 −2i₁ − 3i₂ + 6i₃ = 6

Step 4: Solve the Equations: Here we are using Cramer’s rule to solve the equations.

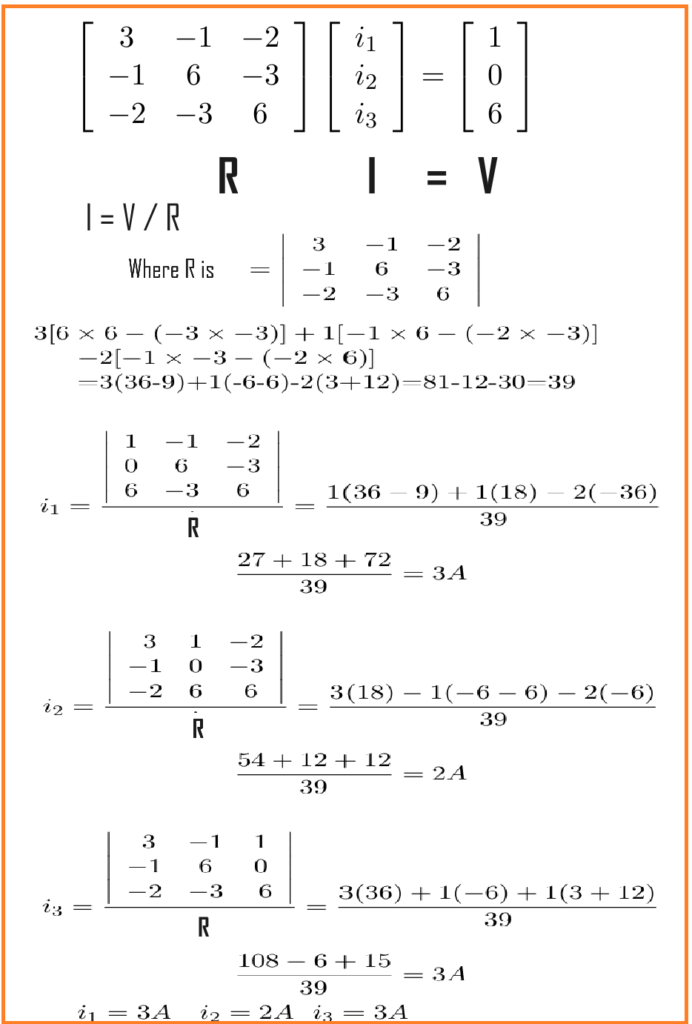
Mesh Current Analysis involves labeling loops, creating matrices for voltages and resistances, and solving a matrix equation to find the currents. This method simplifies circuit analysis, reducing the amount of math required.