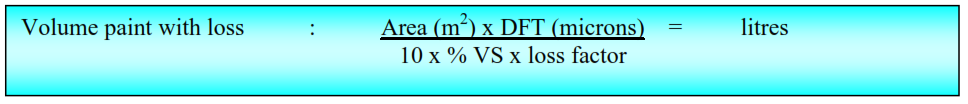Often there is a lot of confusion when paint consumption is going to be calculated. Various paint manufacturers arrive at different volumes, and thereby also different costs, when giving quotations for a specific job. It is not possible to find the true coating costs without a uniform method of calculating. The following requisites are necessary for calculating the correct quantity and coating costs:
a. Percent volume solids of the products
b. Dry film thickness recommended
c. Number of coats
d. Surface area and condition of area to be coated
e. Method of application
f. Cost per liter paint
g. Loss factor
CALCULATIONS OF PAINT CONSUMPTION
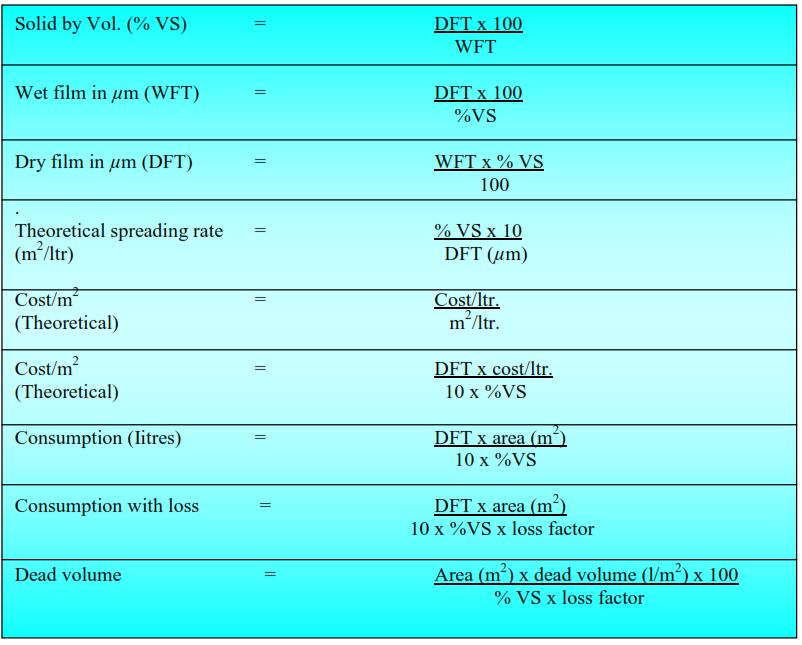
Percent Volume Solids (% VS)
Percent volume solids is the volume percentage of solid material in the coating. The remaining volume percentage consists of volatile solvents or thinners. The solids are the film-forming portions of the coating that will remain on the surface after drying or curing. Percent volume solids is the only true basis for calculating paint volumes required. Solids content quoted by weight is a figure that for most coatings and applications is meaningless. The percent volume solids is found in the technical data sheet (TDS), but can also be found or verified by standardized tests.
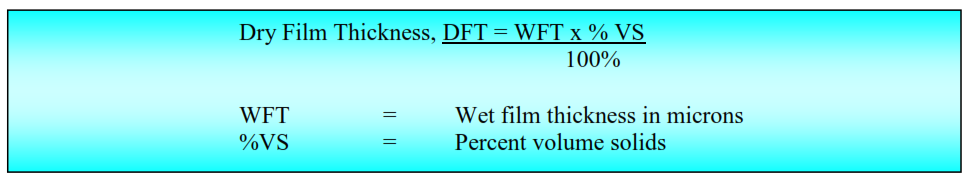
Dry Film Thickness (DFT)
The dry film thickness (DFT) of an applied coating can be determined if the percent volume solids (% VS) and the wet film thickness (WFT) are known. This information can be obtained from the manufacturer’s Technical Data Sheets. The equation for calculating the dry film thickness is:
Example 1:
A coating is to be applied at wet film thickness of 250 microns, and the volume solid is 50 percent.
The equation becomes:
Wet film thickness (WFT)
The wet film thickness can be determined if the percent volume solids (% VS) and the dry film thickness (DFT) are known. This information can also be obtained from the manufacturers Technical Data Sheets. The equation for calculating the WFT is:
Example 2:
A coating is to be applied at a dry film thickness of 100 microns, and the volume solid is 65%.
WFT= 100 microns x 100%/65% = 153.9 microns = 154 microns
The coating must be applied in a WFT of 154 microns since 35% of the paint is solvents that will evaporate.
Wet film thickness after thinning
If the coating is thinned 20% to adjust the viscosity for application the solid by volume will change, it decreases. The new volume percent solids can be calculated or found in a table.
Example 3:
If one liter of a coating with 65% volume solid is thinned 20%, the total volume of the coating will increase to 1.2 liters. The new percentage by volume of solid is:
VS after thinning: % VS prior to thinning / New volume of paint = 65% /1.2 litres= 54%
The new WFT, to get a DFT of 100 microns after adding 20%, is:
New WFT: 100 microns x 100% /54% = 185 microns
The coating must be applied in a WFT of 185 microns, since 46% of the paint in the tin is solvents that evaporate.
Example 4:
Another way of calculating the new WFT after thinning is given by the formula:
New WFT: DFT x (100 + % added thinner)/% VS = 100 microns x 120/65% = 185 microns
See also the table “Volume solids after thinning”.
Theoretical spreading rate of a coating
The theoretical spreading rate (m2 /litre) is the area covered by one litre of paint for a given dry film thickness. Thus, the spreading rate can be determined if the percent volume solid and the desired dry film thickness are known. The equation for calculation is:
Theoretical spreading rate, m2/litre = % VS x 10/DFT
How to arrive at the constant 10 is shown below.
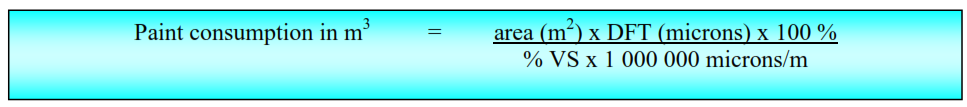
8.7 Consumption of paint, theoretical value
The theoretical consumption of a coating can be determined if the area (m2), the percent volume
solid (% VS) and the desired dry film thickness (DFT) are known. The equation to get the
theoretical consumption is:
The constant 10 is a correction factor for the different units included in the formula and derives
from the following:
First, let us calculate the paint consumption in m3. Here we have to include the correlation factor
for transforming from meters to microns. We know that 1 m = 1.000.000 microns, and get the
following equation:
However, we would like to order the paint in liters and not in m3. Then, we have to convert by
introducing that 1 m3
= 1000 liters, and we get the equation:
Paint consumption = Area x DFT / % VS x 10
giving the formula as below:
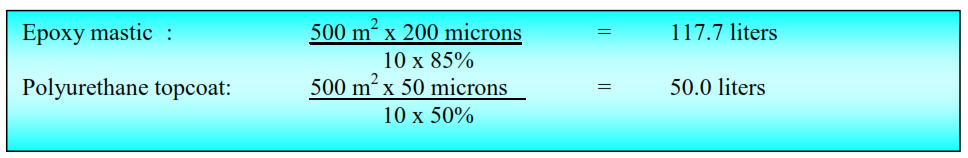
Example 5:
The outside of a tank is to be painted. The total area is 500 m2. The following paint system is to be
applied by airless spray:
How much paint do we need theoretically for the job?
8.8 Paint consumption with loss
The above example assumes that there is no loss of paint during the job and that every drop of paint
is applied to the surface. In a real situation some of the paint will be lost:
The coating may miss target due to strong wind, very complex geometry of the structure or by poor application technique.
Overspray due to complex geometry of the structure or by poor application technique.
Some material will be left in the pump, the tins or in the container.
Dead volume
Such paint losses are usually in the range of 25-40%, but may accumulate to as high as 50% of the total theoretical volume. Loss of paint must always be included when the total paint consumption is calculated. Further, it is important to calculate the volume of paint to order in the correct manner. If
the theoretical amount of paint needed is 100 liters and the loss is 40%, only 60% of the actually paint is left on the surface after painting. The loss factor is 0.6. The equation for calculation is:
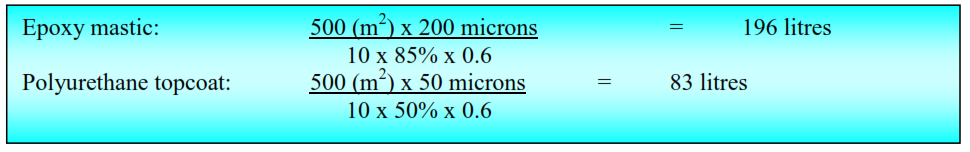
Example 6:
The same figures as for Example 5 are used, but now a loss of 40% (loss factor of 0.6) is included. The correct amount of print to order will be:
Paint consumption with Loss- and Dead Volume factor
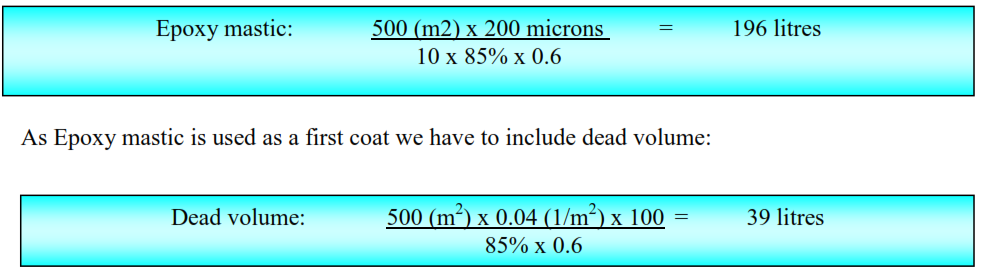
Blast cleaning will increase the surface roughness and thereby increase the surface area. The roughness will depend on the abrasive used. However, the consequence is that more paint is needed to cover the increased area. The ‘dead volume’ is the amount of paint needed to fill in for the roughness (profile) on the blast-cleaned steel (from the bottom to the top of the valley). The amount of paint (1/m2 ) needed to fill in the roughness is indicated below:
NB! The “dead volume” factor only affects the paint consumption for the primer or the first coat of a paint system.
Example 7:
Here example 6 is taken one step further. The loss is 40%. The surface of 500 m2 is blast cleaned to Sa 21/2 with a roughness of 60 microns. A roughness of 60 microns gives a dead volume of 0.04l/m (see table above).
The paint consumption with loss, calculated in example 6, is:
The total need for Epoxy mastic is: 39 liters + 196 liters = 235 liters. As the dead volume will have no influence on the topcoat the volume of the Polyurethane is the same as in example 6: